Answer:
Option B) -0.23
Explanation:
We are given the following information in the question:
Mean, μ = 108
Standard Deviation, σ = 28.15
Formula:

We are given x = 101.67
We have to find the corresponding z-score.
Putting values, we get,
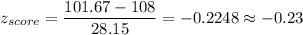
Thus, the correct answer is
Option B) -0.23