Answer:5
Step-by-step explanation:
Given
First Plate has a charge of +Q and area A
Second Plate has a charge of -3 Q and area A
We Know electric Field due to sheet charge is given by
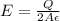
Where Q=charge over the Plate
A=Area of plate
 = Permittivity of free space
= Permittivity of free space
Electric Field Due to Positive charge will always be away from it while for negative charge it is towards it.
Net Electric Field at a point between between the Plates is the superimposition of two electric with direction

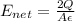
Net electric Field is towards the negative charged plate