Answer:

Explanation:
The given square root function is
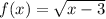
The domain refers to all values of x that will make this square root function defined.
We know that negative values will not be defined for the square root function therefore the expression under the root should be greater or equal to zero.
Hence the inequality that can be used to find the domain of f(x) is
