Question

Mathematics achievement test scores for 300 students were found to have a mean and a variance equal to 600 and 3600, respectively.

(a) If the distribution of test scores was mound-shaped, approximately how many of the scores would fall into the interval 540 to 660? (Round your answer to the nearest whole number.)
(b) Approximately how many scores would be expected to fall into the interval 480 to 720? (Round your answer to the nearest whole number.)

Answer 1

(a) Approximately 205 students scored between 540 and 660.

(b) Approximately 287 students scored between 480 and 720.

Explanation:

A mound-shaped distribution is a normal distribution since the shape of a normal curve is mound-shaped.

Let X = test score of a student.

It is provided that
`X\sim N(\mu = 600, \sigma^(2) = 3600)`.

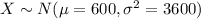(a)

The probability of scores between 540 and 660 as follows:

`P(540\leq X\leq 660)=P((540-600)/(√(3600) )\leq (X-600)/(√(3600) )\leq (660-600)/(√(3600) ))\\=P(-1 \leq Z\leq 1)\\= P(Z\leq 1)-P(Z\leq -1)\\=0.8413-0.1587\\=0.6826`

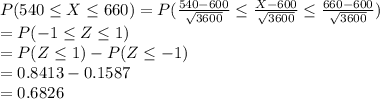
Use the standard normal table for the probabilities.

The number of students who scored between 540 and 660 is:

300 × 0.6826 = 204.78 ≈ 205

Thus, approximately 205 students scored between 540 and 660.

(b)

The probability of scores between 480 and 720 as follows:

`P(480\leq X\leq 720)=P((480-600)/(√(3600) )\leq (X-600)/(√(3600) )\leq (720-600)/(√(3600) ))\\=P(-2 \leq Z\leq 2)\\= P(Z\leq 2)-P(Z\leq -2)\\=0.9772-0.0228\\=0.9544`

Use the standard normal table for the probabilities.

The number of students who scored between 480 and 720 is:

300 × 0.9544 = 286.32 ≈ 287

Thus, approximately 287 students scored between 480 and 720.