Answer:
The length of each side of the larger square is 8 centimeter.
Explanation:
Given:
The combined area of two squares is 80 square centimeters. Each side of one square is twice as long as a side of the other square.
Now, to find the length of each side of the larger square.
Let
 be the length of the smaller square.
be the length of the smaller square.
So, the length of the larger square =

Now, we find the areas of the square by putting formula:
The area of smaller square = length²
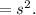
The area of larger square = (length)²

As, given:
The combined area of two squares is 80 square centimeters.
According to question:

So, dividing both sides by 5 we get:
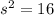
Using square root on both sides we get:
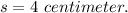
And, to get the length of each side of the larger square we substitute the value of
 :
:

The length of larger square = 8 centimeter
Therefore, the length of each side of the larger square is 8 centimeter.