Answer:
Options B and C are correct choices.
Explanation:
We have been given 4 pairs of ratios. We are asked to choose the pairs that form a proportion.
We know that two quantities are proportional if their ratios are same.
Let us check our each pair.
A. One sixth and four over twenty.
Express as fractions and equate:
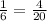
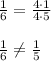
Since ratio is not same, therefore, this pair is not proportional.
B. Seven ninths and twenty eight over thirty six.
Express as fractions and equate:
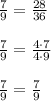
Since ratio is same, therefore, this pair is proportional.
C. Fourteen over eighteen and twenty one over twenty seven.
Express as fractions and equate:
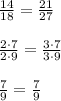
Since ratio is same, therefore, this pair is proportional.
C. Thirty over eighty and six over eighteen.
Express as fractions and equate:
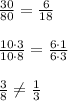
Since ratio is not same, therefore, this pair is not proportional.