Answer:
The maximum load the rod can take before it starts to permanently elongate = = 6086.84 lbf or 27075.59 N
The maximum energy that the rod would store =2.536 ft·lbf or 3.439 J
Step-by-step explanation:
We list out the variables to the question as follows
Rod diameter = 0.5 in
Length of rod = 5 in
Young's modulus of elasticity for the material = 15.5 Msi
The maximum load rthe rd can take before it starts to permanently elongate is the yield stress and it can vbe calclulated by applyng the 0.2% offset rule by taking the yeild strain to be equal to 2%
Thus Young's Modulus at yield point =
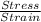 so that the yield stess is
so that the yield stess is
Yield stress = strain × Young's Modulus
= 2/100 × E = 0.002 × 15.5 Msi = 0.031 Msi = 31 ksi
The maximum load that the rod would take before it starts to permanently elongate = F = Stress×Area
where area = π·D²/4 = 0.196 in²
F = 31 kSi × 0.196 in² = 6086.84 lbf or 27075.59 N
To calculate the strain energy stored in the rod we apply the strain energy formula thus
U = V×σ²/2·E
To calculate the volume we have V = L ×π·D²/4 =5 in × 0.196 in² = 0.98 in³
then U = 0.98 × (31000)²/(2·15.5×10⁶)
= 30.43 in³·psi = 2.536 ft·lbf
or 3.439 J
The maximum energy that the rod would store = 2.536 ft·lbf