Question

A mechanical engineer launched an arrow with a speed of 800.0 m/s at an angle of 75.0 degree to the horizontal . If it landed on a target 450.0 m away at the same height from which it was fired , for how long was the arrow in the air ?

Answer 1

Answer: 2.17 s

Step-by-step explanation:

The described situation is related to projectile motion (also known as parabolic motion). So, this kind of motion has a vertical component and a horizontal component; however, in this case we will only need the equation related to the horizontal displacement
`x`:

`x=V_(o)cos \theta t`

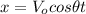
Where:

`x=45 m` is the arrow's horizontal displacement

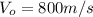`V_(o)=800 m/s` is the arrow's initial velocity

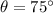`\theta=75\°` is the angle

`t` is the time the arrow is in the air

Isolating
`t`:

`t=(x)/(V_(o)cos \theta)`

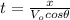
Solving with the given data:

`t=(45 m)/(800 m/s cos(75\°))`

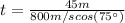
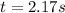`t=2.17 s` This is the time the arrow is in the air