Question

A 124-kg balloon carrying a 22-kg basket is descending with a constant downward velocity of 20.0 m/s. A 1.0-kg stone is thrown from the basket with an initial velocity of 15.0 m/s perpendicular to the path of the descending balloon, as measured relative to a person at rest in the basket. That person sees the stone hit the ground 5.00 s after it was thrown. Assume that the balloon continues its downward descent with the same constant speed of 20.0 m/s. (a) How high is the balloon when the rock is thrown? (b) How high is the balloon when the rock hits the ground? (c) At the instant the rock hits the ground, how far is it from the basket? (d) Just before the rock hits the ground, find its horizontal and vertical velocity components as measured by an observer (i) at rest in the basket and (ii) at rest on the ground.

Answer 1

a)-296 m/s

b)-176 m

c) 198 m

Step-by-step explanation:

given data:

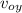`v_(oy)\\`=-20 m/s

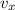`v_(x)`= 15 m/s

t = 6 s

To find

a)
`H_(1)`

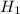
b)
`H_(2)`

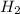
c) d

d) find the velocity of observer at rest in basket and rest on ground

a) according to kinematic equation the displacement is given by:

`H_(1)`=
`v_(oy)t\\`-
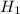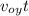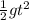`(1)/(2) gt^(2)`..............(1)

putting values in 1

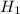`H_(1)`=-296 m/s

negative sign is due to direction and also we choose to take off point to be the origin

b) while the stone was travelling
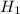`H_(1)` for 6 s so the ballon was also travelling displacement
`H_(b)` with
`v_(oy)\\`

`H_(b)` =
`v_(oy)t\\`

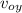
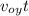
=-6*20

=-120 m

the height above the earth is given by

`H_(2)`=
`H_(1)`-
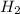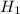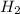`H_(2)`= -176 m

c) the horizontal displacement is given by

x=
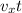`v_(x)t\\`=15*6=90 m

so the distance between balloon and stone is

d=
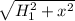`\sqrt{H_(1)^(2)+x^(2) }`=198 m

d) the velocity component of the balloon :

1) relative to person rest in the balloon are given by

The vertical component:

`v_(yr) =v_(by) +v_(py)`=
`(-gt)-0=9.8*6=-58.8 m/s`

The horizontal component:

`v_(xr) =v_(bx) +v_(px)= 15-0=15m/s`

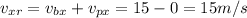
2) relative to person rest in the earth are given by

The vertical component:

`v_(yr) =v_(by) -v_(py)=(-gt)-20=9.8*6-20=-78.8 m/s`

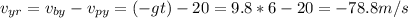
The horizontal component:

`v_(xr) =v_(bx) -v_(px)= 15-0=15m/s`