Question

Suppose that the cost of electrical energy is $0.15 per kilowatt hour and that your electrical bill for 30 days is $80. Assume that the power delivered is constant over the entire 30 days. What is the power in watts? If a voltage of 120 V supplies this power, what current flows? Part of your electrical load is a 60-W light that is on continuously. By what percentage can your energy consumption be reduced by turning this light off?

Answer 1

a) 740 W b) 6.2 A c) 8.1%

Step-by-step explanation:

We need first to get the total energy spent during the 30 days, that can be calculated as follows:

1 month = 30 days = 720 hr

If the total cost amounts $80 (for 720 hr), and the cost per kwh is 0.15, we have:

80 $/mo = 0.15 $/Kwh*x Kwh/mo

Solving for the total energy spent in the month:

`x (kwh/mo) = (80)/(0.15) = 533.3 kwh`

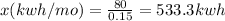
Assuming that the power delivered is constant over the entire 30 days, as power is the rate of change of energy, we can find the power as follows:

`P = (E)/(t) = (533.3 kWh)/(720 h) = 0.74 kW = 740 W`

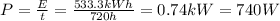
b) If the power is supplied by a voltage of 120 V, we can find the current I as follows:

`I =(P)/(V) =(740W)/(120V) = 6.2A`

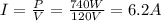
c) If part of the electrical load is a 60-W light, we can substract this power from the one we have just found, as follows:

P = 740 W - 60 W = 680 W

The new value of the energy spent during the entire month will be as follows:

E = 0.68 kW*24(hr/day)*30(days/mo) = 490 kWh/mo

The reduction in percentage regarding the total energy spent can be calculated as follows:

ΔE =
`(533.3-490)/(533.3) = 0.081*100= 8.1%`

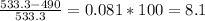
⇒ ΔE(%) = 8.1%

`%(E) =(533.3-490)/(533.3) = 0.081 * 100 = 8.1%`