Answer:
On moon time period will become 2.45 times of the time period on earth
Step-by-step explanation:
Time period of simple pendulum is equal to
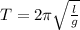 ....eqn 1 here l is length of the pendulum and g is acceleration due to gravity on earth
....eqn 1 here l is length of the pendulum and g is acceleration due to gravity on earth
As when we go to moon, acceleration due to gravity on moon is
 times os acceleration due to gravity on earth
times os acceleration due to gravity on earth
So time period of pendulum on moon is equal to
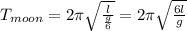 --------eqn 2
--------eqn 2
Dividing eqn 2 by eqn 1
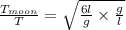
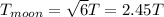
So on moon time period will become 2.45 times of the time period on earth