Answer: There are 1540 different orders.
Explanation:
- The number of ways to arrange n things where 'a' things are alike and 'b' things are a like and so on...
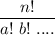
Given : Total items = 22
Defective items = 3
Not defective items = 22-3 = 19
Then, the number of different orders can the 22 items be arranged if all the defective items are considered identical and all the non-defective items are identical of a different class :
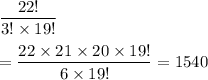
Hence, there are 1540 different orders.