The concept of equilibrium which is useful when dealing with forces is states that a body on which exactly balanced forces act has no net force acting on it and the body is said to be in equilibrium
The weight of the suspended block E is approximately 18.33-lb
The reason why the value of the weight is correct is as follows:
The given parameters are;
The length of the chord = 4-ft.
The weight of the block D = 10-lb
The horizontal distance between pin A and C = 1 ft.
The length of the segment of the string s when the system is in equilibrium = 1.5 ft.
Required:
To determine the weight of the suspended block E, if the system is in equilibrium
Solution:
The tension in the cord, T = The weight of block D = 10-lb
By equilibrium of forces, we have;
E = 2 × T × cos(θ)
Where:
E = The weight of the block located at E
θ = The angle between segment CB and the vertical
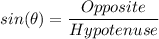
The length of the opposite side to θ = 1 ft./2 = 0.5 ft.
The length of the hypotenuse side =

Therefore;
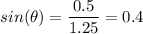
θ ≈ 23.58°
E = 2 × 10 × cos(23.58°) ≈ 18.33 lb.
The weight of the suspended block E ≈ 18.33 lb.
Learn more about the equilibrium of forces here: