Answer:
The average velocity is 22.
Explanation:
It is given that the function s(t) represents the position of an object at time t moving along a line.
If a position function is s(t), then the average velocity of the object over the interval of time [a,b] is
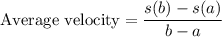
The average velocity of the object over the interval of time [1,3] is
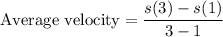
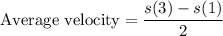
It is given that s(1)=105 and s(3)=149. Substitute these values in the above formula.
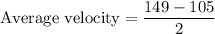
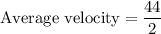
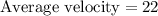
Therefore, the average velocity is 22.