Question

Equations, graphs, Slopes and y-intercepts : application

Answer 1

`y=(1)/(2)x-5`

Step-by-step explanation:

Slope-intercept form: y = mx + b

m = slope

`m=(y_2-y_1)/(x_2-x_1)`

To find slope, we use points on the line.

Here, I will be suing (-6, -8) and (8, -1)

`m=(1-(-8))/(8-(-6)) \\\\m=(-1+8)/(8+6) \\\\m=(7)/(14)\\\\m=(1)/(2)`

`y=mx+b`

`y=(1)/(2) x+b\\`

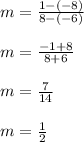
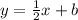
Now, we use either of our points (-6, -8) OR (8, 1) to find b.

I will be using (8, -1):

`y=(1)/(2)x+b\\\\-1=(1)/(2)(8)+b\\\\-1=4+b\\\\-4-4\\\\-5=b`

`y=(1)/(2)x+b== > y=(1)/(2)x-5`

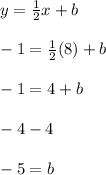
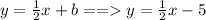
Check your answer manually: (-6, -8)

`y=(9)/(14)x-(29)/(7)\\\\-8=(9)/(14)(-6)-(29)/(7)\\\\-8=(9)/(7)(-3)-(29)/(7)\\\\-8=-(27)/(7)-(29)/(7)\\\\-8=-(56)/(7)\\\\-8=-8`

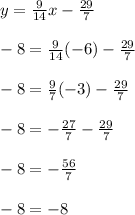
This statement is correct.

*You can also view the attached graph to verify the answer, meaning that those two points should lie on the same line.*

Hope this helps!

Answer 2

`\sf y= (1)/(2)x-5`

Step-by-step explanation:

• coordinates taken: (0, -5), (6, -2)

slope:

`\rightarrow \sf (y_2-y_1)/(x_2-x_1)`

`\rightarrow \sf (-2--5)/(6-0)`

`\rightarrow \sf (1)/(2)`

equation in slope intercept form:

• y = m(x) + b [ where "m is slope", "b is y-intercept" ]

`\sf y= (1)/(2)x-5`