Question

John randomly picks a number from 1 to 5 (both inclusive) and a letter from A to E (both inclusive). What is the probability that he picks 2 and E? Select the correct option. Select one: a. 1/25 b. 1/30 c. 1/4 d. 1/5

Answer 1

Answer: a. 1/25

P(2∩E) = 1/25

Explanation:

Given;

The two set of numbers and letters

Set 1 = { 1,2,3,4,5}

Set 2 = { A,B,C,D,E}

The probability that he picks 2 and E equals the probability of picking 2 from set 1 multiplied by the probability of picking E from set 2.

Let P(2) represent the probability of picking 2 from set 1 ,

P(E) represent the probability of picking E from set 2 and

P(2∩E) represent the probability of picking 2 from set 1 and E from set 2.

P(2∩E) = P(2) × P(E) .....1

P(2) = N(2)/N(T)

N(2) = 1 (there is only one 2 in the set)

N(T) = 5 (total number of elements in set 1)

P(2) = 1/5

P(E) = N(E)/N(T)

P(E) = 1/5

Substituting P(E) and P(2) into equation 1

P(2∩E) = 1/5 × 1/5

P(2∩E) = 1/25

Answer 2

`P(A \cap B) = P(A) *P(B) =(1)/(5) (1)/(5)= (1)/(25)`

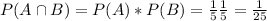
So then the best option would be:

a. 1/25

Explanation:

For this case we assume that the sample space for the numbers is :

`S_1= [A,B,C,D,E]`

And the sample space for the numbers is:

`S_2 =[1,2,3,4,5]`

Both sampling spaces with a size of 5.

We define the following events:

A="We select a 2 from the numbers"

B= "We select a E from the letters"

We can find the individual probabilities for each event like this:

`P(A)= (1)/(5)`

`P(B) = (1)/(5)`

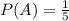
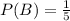
And assuming independence we can find the probability required like this:

`P(A \cap B) = P(A) *P(B) =(1)/(5) (1)/(5)= (1)/(25)`

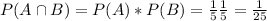
The last probability is the probability of obtain obtain a 2 AND an E

So then the best option would be:

a. 1/25