Question

A planet exerts a gravitational force of magnitude 9e22 N on a star. If the planet were 2 times closer to the star (that is, if the distance between the star and the planet were 1/2 what is is now), what would be the magnitude of the force on the star due to the planet?

Answer 1

To solve this problem we will use the related concepts in Newtonian laws that describe the force of gravitational attraction. We will use the given value and then we will obtain the proportion of the new force depending on the Radius. From there we will observe how much the force of attraction increases in the new distance.

Planet gravitational force

`F_p = 6*10^(22)N`

`F_p = (GMm)/(R^2)`

`F_p = 9*10^(22)N`

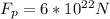
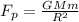
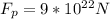
Distance between planet and star

`r = (R)/(2)`

Gravitational force is

`F = (GMm)/(r^2)`

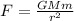
Applying the new distance,

`F = (GMm)/(((R)/(2))^2)`

`F = 4(GMm)/(R^2)`

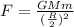
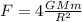
Replacing with the previous force,

`F = 4F_p`

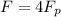
Replacing our values

`F= 4(9*10^(22)N)`

`F = 36*10^(22)N`

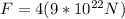
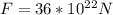
Therefore the magnitude of the force on the star due to the planet is
`36*10^(22)N`