Answer:
Perimeter of the kite is 16.2 units rounding of to the nearest tenth.
Explanation:
Since, WXYZ is a kite, two separate pairs of repeated sides are congruent
This means,
WX=XY
WZ=ZY
∴, perimeter of kite WXYZ is = 2 (WX + WZ) Bar
(WX)Bar =
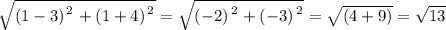
(WZ)Bar =
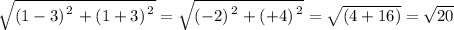
Hence, Perimeter P =
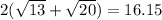 ≈ 16.2 Units
≈ 16.2 Units