Answer:
There are 2,480,721,300,000,00 ways to paint this set.
Explanation:
We have that:
A set of 27 elements, of which:
7 are painted white
6 are painted old gold
2 are painted blue
7 are painted yellow
5 are painted green
How many ways are there to paint?
A single change in the set, for example, element 0 exchanged with element 1, means we have a new way. So we use the permutations formula to solve this problem:
Permutations
Permutations of a set of x elements divided into sets of size w,y,z.
The number of ways is:
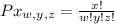
In this problem, we have that:
A set of 27 divided into sets of 7,6,2,7,5. So

There are 2,480,721,300,000,00 ways to paint this set.