Answer with Step-by-step explanation:
We are given that function f(x) which is quadratic function.
x -intercept of function f(x) at (-1,0) and (-3,0)
x-Intercept of f means zeroes of f
x=-1 and x=-3
Range of f =[-4,
 )
)
g(x)=
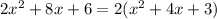
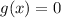
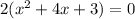

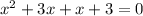
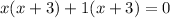
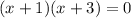
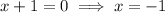

Therefore, x-intercept of g(x) at (-1,0) and (-3,0).
Substitute x=-2
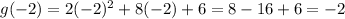
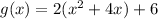

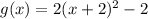
By comparing with the equation of parabola

Where vertex=(h,k)
We get vertex of g(x)=(-2,-2)
Range of g(x)=[-2,
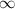 )
)
Zeroes of f and g are same .
But range of f and g are different.
Range of f contains -3 and -4 but range of g does not contain -3 and -4.
f and g are both quadratic functions.