Question

You collect a random sample of size n from a population and calculate a 90% confidence interval. Which of the following strategies would produce a new confidence interval with an increased margin of error?A. Use an 80% confidence level.B. Use the same confidence level, but compute the interval n times. Approximately 10% of these intervals will be larger.C. Use an 85% confidence level.D. Decrease the sample size.E. Nothing can guarantee that you will obtain a larger margin of error. You can only say that the chance of obtaining a larger interval is 0.10.

Answer 1

D. Decrease the sample size.

Explanation:

How we find the margin of error of a conficence interval?

We have that to find our
`\alpha` level, that is the subtraction of 1 by the confidence interval divided by 2. So:

So a 90% confidence interval

`\alpha = (1-0.9)/(2) = 0.05`

Now, we have to find z in the Ztable as such z has a pvalue of
`1-\alpha`.

So it is z with a pvalue of
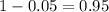`1-0.05 = 0.95`, so
`z = 1.645`

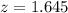
95% confidence interval

`\alpha = (1-0.95)/(2) = 0.025`

So it is z with a pvalue of
`1-0.025 = 0.975`, so
`z = 1.96`

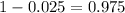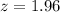
So, as the confidence level increases, so does the value of z.

Now, find the margin of error M as such

`M = z*(\sigma)/(√(n))`

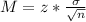
In which
`\sigma` is the standard deviation of the population and n is the size of the sample.

From this, we have that as z increases, so does the margin of error. So as the confidence level increases, so does the margin of error.

Also, as the size of the sample increases, the margin of error decreases. And as the size of the sample decreases, the margin of error increases.

We want to increase the margin of error. So the correct answer is:

D. Decrease the sample size.