Answer:
Given Parallelogram EFGH
EG bisects HF and HF bisects EG, if and only if both the diagnols have same mid point.
Explanation:
Step 01:
Let
E be the point (a,b)
F be the point (a',b)
G be the point (a',b')
H be the point (a,b')
Step 02:
Now find mid points of EG and HF
mid point of EG = (
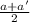 ,
,
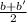 ) and
) and
mid point of HF = (
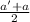 ,
,
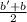 )
)
Since addition is commutative, and they have the same mid-point, so they bisect each other.