Answer: 3.41 s
Step-by-step explanation:
Assuming the question is to find the time
 the ball is in air, we can use the following equation:
the ball is in air, we can use the following equation:

Where:
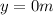 is the final height of the ball
is the final height of the ball
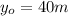 is the initial height of the ball
is the initial height of the ball
 is the initial velocity of the ball
is the initial velocity of the ball
 is the time the ball is in air
is the time the ball is in air
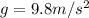 is the acceleration due to gravity
is the acceleration due to gravity

Then:
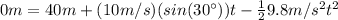
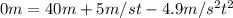
Multiplying both sides of the equation by -1 and rearranging:

At this point we have a quadratic equation of the form
 , which can be solved with the following formula:
, which can be solved with the following formula:
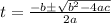
Where:
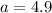
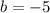
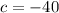
Substituting the known values:
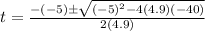
Solving the equation and choosing the positive result we have:
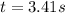 This is the time the ball is in air
This is the time the ball is in air