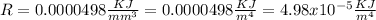Answer:
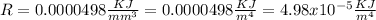
Explanation:
For this case we have the following value:
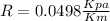
We can convert this first to
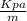 like this:
like this:
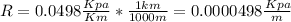
Now we use the fact the the pressure is defined as
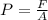 , whre P is the pressure, F the force and A the area, so then
, whre P is the pressure, F the force and A the area, so then
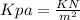 and then we can replace this:
and then we can replace this:
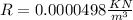
Now from definition of work we know that
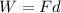 where W is the work, F the force and d the distance, so then is equivalent
where W is the work, F the force and d the distance, so then is equivalent
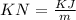
And if we replace this into the equation we got: