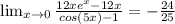Answer:
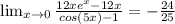
Explanation:
Notice that
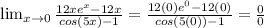 , which is in indeterminate form, so we must use L'Hôpital's rule which states that
, which is in indeterminate form, so we must use L'Hôpital's rule which states that
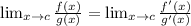 . Basically, we keep differentiating the numerator and denominator until we can plug the limit in without having any discontinuities:
. Basically, we keep differentiating the numerator and denominator until we can plug the limit in without having any discontinuities:
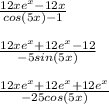
Now, plug in the limit and evaluate:

Thus,