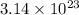Question

How many moles of ions form when 51.0 g of calcium iodide is dissolved in water? how many ions form?

Answer 1

Answer : The number of moles of ions form are,
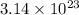`3.14* 10^(23)` and there are 3 ions formed.

Explanation :

First we have to calculate the moles of calcium iodide.

`\text{Moles of }CaI_2=\frac{\text{ given mass of }CaI_2}{\text{ molar mass of }CaI_2}`

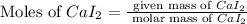
Molar mass of calcium iodide = 293.9 g/mol

Mass of calcium iodide = 51.0 g

`\text{Moles of }CaI_2=(51.0g)/(293.9g/mole)=0.174moles`

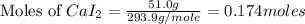
Now we have to calculate the number of moles of ions.

As we know that when calcium iodide dissolved in water then it dissocites to give calcium ion and iodide ion.

The balanced chemical reaction will be:

`CaI_2(aq)\rightarrow Ca^(2+)(aq)+2I^(-)(aq)`

From this we conclude that there are 3 ions formed.

As, 1 mole of calcium iodide dissociate to give
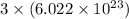`3* (6.022* 10^(23))` number of ions

So, 0.174 mole of calcium iodide dissociate to give
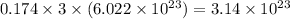`0.174* 3* (6.022* 10^(23))=3.14* 10^(23)` number of ions

Thus, the number of moles of ions form are,
`3.14* 10^(23)`