Question

In an aqueous solution of a certain acid the acid is 4.4% dissociated and the pH is 3.03. Calculate the acid dissociation constant Ka of the acid. Round your answer to 2 significant digits.

Answer 1

4.1x10⁻⁵

Step-by-step explanation:

The dissociation of an acid is a reversible reaction, and, because of that, it has an equilibrium constant, Ka. For a generic acid (HA), the dissociation happens by:

HA ⇄ H⁺ + A⁻

So, if x moles of the acid dissociates, x moles of H⁺ and x moles of A⁻ is formed. the percent of dissociation of the acid is:

% = (dissociated/total)*100%

4.4% = (x/[HA])*100%

But x = [A⁻], so:

[A⁻]/[HA] = 0.044

The pH of the acid can be calcualted by the Handersson-Halsebach equation:

pH = pKa + log[A⁻]/[HA]

3.03 = pKa + log 0.044

pKa = 3.03 - log 0.044

pKa = 4.39

pKa = -logKa

logKa = -pKa

Ka =
`10^(-pKa)`

Ka =
`10^(-4.39)`

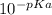
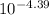
Ka = 4.1x10⁻⁵