The complete question is:
A geometric sequence is defined recursively by
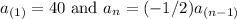
(a) Write out the first four terms of this sequence.
(b) Is the 9th term of this sequence larger or smaller than 1/10? Show the calculation that you use to determine your answer.
Answer:
- The 9th term is larger than 1/10.
Step-by-step explanation:
You are given the first term,
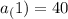 , and the recursive formula
, and the recursive formula
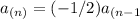
Thus, you can find the sequence of terms by multiplying each term by the constant ratio, (-1/2).
(a) First four terms of the sequence
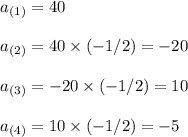
Those are the first four terms.
(b) 9th term
You can write the explicit formula of the sequence as:
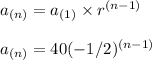
Thus, for the 9th term, n = 9, and the term is:
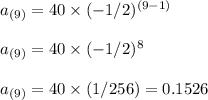
Since 1/10 = 0.1, and 0.1 < 0.1526, the 9th term is larger than 1/10.