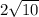The distance between the two points is
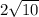
Explanation:
The given coordinates is
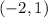 and
and

To find the distance between two points, let us use the distance formula,
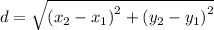 where
where
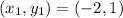 and
and
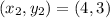
Thus, substituting the values in the formula, we get,
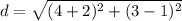
Simplifying the expression,
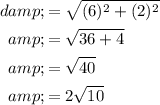
Thus, the distance between the two points is