Question

A 115 kg astronaut on a space walk pushes against her space capsule that has mass 2250 kg. The astronaut accelerates 1.50 m/s2, 0. (a) Find the force exerted on the astronaut. (b) Find the force exerted on the capsule. (c) Find the acceleration of the capsule

Answer 1

Step-by-step explanation:

Given that,

Mass of the astronaut, m = 115 kg

Mass of the capsule, M = 2250 kg

The acceleration of the astronaut,
`a=1.5\ m/s^2`

(a) The force exerted on the astronaut is given by :

F = ma

`F=115\ kg* 1.5\ m/s^2`

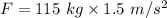
F = 172.5 N

(b) The force force exerted on the capsule is equal to the force exerted on the astronaut as per Newton's third law of motion. So, the force exerted on the capsule is 172.5 N.

(c) The acceleration of capsule is given by :

`a'=(F)/(M)`

`a'=(172.5)/(2250)`

`a'=0.076\ m/s^2`

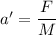
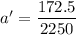
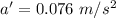
Hence, this is the required solution.