Answer:
A. x = -4 + 3t, y = 3 - 4t: - 2 lessthanorequalto t lessthanorequalto 3
Explanation:
Given that a line in two dimension passes through (-4,3) and (2,-5) oriented in the direction of increasing x
We can write the line equation in parametric form as
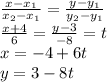
The values of t when x=-4 is 0 and when x =2 is 1
So t varies from 0 to 1
If instead of t we give t' say which is t +2
then we have
t he parametric equations as
x =-4+3t and y = 3-4t
For x=-4, t =2 and for x = 2 , t =3
So option A is right.