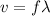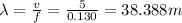Question

A mass of 13.9 kg bounces up and down from a spring with constant 9.3 N/m. Toward the bottom of its motion the mass dips into a pool of water and comes back out. The wave created by this process travels away at 5 m/s. What is the associated wavelength of this water wave measured in meters?

Answer 1

Wavelength will be 38.388 m

Step-by-step explanation:

We have given mass m = 13.9 kg

Spring constant K= 9.3 N/m

Velocity v = 5 m /sec

Angular frequency is given by
`\omega =\sqrt{(k)/(m)}`

So
`\omega =\sqrt{(9.3)/(13.9)}=0.817`

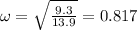
Now we have to find frequency for further calculation

So frequency will be equal to
`f=(\omega )/(2\pi )=(0.817)/(2* 3.14)=0.130Hz`

Now we have to find wavelength, it is ratio of velocity and frequency

There is a relation between frequency velocity and wavelength

`v=f\lambda`

`\lambda =(v)/(f)=(5)/(0.130)=38.388m`

Answer 2

`\lambda_w=0.6509\ m`

Step-by-step explanation:

Given:

• mass oscillating with the spring,
  `m=13.9\ kg`

• spring constant,
  `k=9.3\ N.m^(-1)`

• wave velocity on the water surface,
  `v_w=5\ m.s^(-1)`

Now the angular frequency of the spring oscillation:

`\omega=\sqrt{(k)/(m) }`

`\omega=\sqrt{(9.3)/(13.9) }`

`\omega=0.81796\ rad.s^(-1)`

Now according to the question the wave is created after each cycle of the spring oscillation.

So the time period of oscillation:

`T=(\omega)/(2\pi)`

`T=(0.81796)/(2\pi)`

`T=0.130182\ s`

Now the wave length of the water wave:

`\lambda_w=v_w.T`

`\lambda_w=5* 0.130182`

`\lambda_w=0.6509\ m`