Answer:
From the sample of n= 200 people,
The proportion of people with kids is:
p'= 110/200= 0.55
The confidence interval for population proportion is given by:
![p' - Z_(\alpha /2) \sqrt[]{(p'(1-p'))/(n) } } \leq P\leq p' + Z_(\alpha /2)\sqrt[]{(p'(1-p'))/(n) } }](https://img.qammunity.org/2021/formulas/mathematics/high-school/fwt47fydvsiyr0h3ewwunlxxobyh2b7bl5.png)
![= p' - Z_(\alpha /2) \sqrt[]{(0.55(0.45))/(200) } } \leq P \le p' + Z_(\alpha /2) \sqrt[]{(0.55(0.45))/(200) } }](https://img.qammunity.org/2021/formulas/mathematics/high-school/1auhzapqr7v9dtju1rmhrdbb8pf0hrdo8m.png)
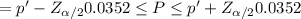
Where P is the population proportion and Z is the critical value at a given level of significance α.