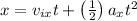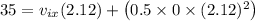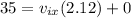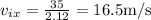The initial horizontal velocity of the soccer ball is 16.5 m/s
Step-by-step explanation:
When we throw a ball, there is a constant velocity horizontal motion and there is an accelerated vertical motion. These components act independently of each other. Horizontal motion is constant velocity motion.
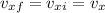
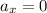 , so
, so
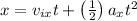 for horizontal motion
for horizontal motion
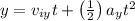 for vertical motion
for vertical motion
Given:
x = 35 m
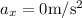
Need to find

y = - 22 m
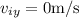
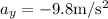 (negative sign indicates downward motion)
(negative sign indicates downward motion)
By substituting all known values, we can solve for 't' value as below
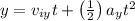
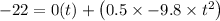
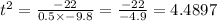
Taking square root, we get t = 2.12 seconds
Now, substitute these to find initial horizontal velocity