Question

Three point charges are located on the positive x-axis of a coordinate system. Charge qı = 1.5 nC is 2.0 cm from the origin, charge q2 = -3.0 nC is 4.0 cm from the origin and charge 93 = 4.5 nC located at the origin. a. What is the magnitude of the net force exerted by the other two charges on charge q1q1q1 = 1.5 nCnC?b. What is the direction of the net force exerted by the other two charges on charge q1q1q1 = 1.5 nCnC?

Answer 1

`F_1=2.53\ 10^(-4) \ N`

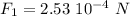
The net force goes to the right

Step-by-step explanation:

Electrostatic Force

Let's consider the situation where 2 point charges q1 and q2 are separated by a distance d. An electrostatic force appears between them whose magnitude can be computed by the Coulomb's formula

`\displaystyle F=(k\ q_1\ q_2)/(d^2)`

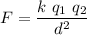
Where k is the constant of proportionality

`k=9.10^9\ Nw.m^2/c^2`

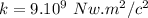
Two equally-signed charges repel each other, two opposite-signed charges attract each other.

We need to find the total net force exerted on q1 by q2 and q3. We're assuming the charges are placed to the right of the origin, so the distribution is shown in the figure below.

Since q3 repels q1, its force goes to the right, since q2 attracts q1, its force goes to the right also, thus the total force on q1 is :

`F_1=F_(31)+F_(21)`

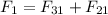
It's directed to the right

Let's compute the individual forces. q3 is separated 2 cm from q1, so d=0.02 m

`\displaystyle F_(31)=(9.10^9\ 4.5\ 10^(-9)\ 1.5\ 10^(-9))/(0.02^2)`

`F_(31)=0.000151875\ N`

`\displaystyle F_(21)=(9.10^9\ 3\ 10^(-9)\ 1.5\ 10^(-9))/(0.02^2)`

`F_(21)=0.00010125\ N`

`F_1=F_(31)+F_(21)=0.000151875\ N+0.00010125\ N=0.000253125 \ N`

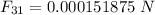
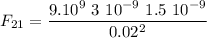
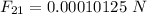
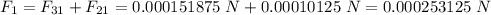
Expressing the force in scientific notation

`\boxed{F_1=2.53\ 10^(-4)\ N}`

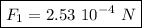
The net force goes to the right

Answer 2

Solution: The magnitude of the net force acting on q₁ is : Fn = 25.312 [N]

and the direction is in the direction of the positive x axis

The electric force between two charges q₁ and q₂, is according to Coulombs´law as:

F₂₁ = K × q₁ × q₂ / d₁₂²

In that equation

F₂₁ is the force exerted by charge q₂ on the charge q₁

• K = 9× 10⁹ [Nm²/C²] Coulomb contant

• q₁ is the charge upon which the force is acting

• q₂ is the force acting over q₁

• d₁₂ is the distance between the charges

It is pretty obvious that F₂₁ = F₁₂, and the force is of rejection if the charges are of the same sign or attraction if they are of opposite signs

Now in this case we calculate F₂₁

F₂₁ = [9×10⁹ × ( 1.5)×10⁻⁹ × (3)×10⁻⁹] /(0.02)²

F₂₁ = 40.5 × 10⁻⁹/ 4 × 10⁻⁴

F₂₁ = 10.125 × 10⁻⁵ [N]

F₂₁ is in the direction of the positive x ( attraction force)

And

F₃₁ = [9×10⁹ × ( 1.5)×10⁻⁹ × (4.5)×10⁻⁹] /(0.02)²

F₃₁ = 15.188× 10⁻⁵ [N]

F₃₁ is in the direction of the positive x ( rejection force)

Then the net force is Fn = 10.125 + 15.188

Fn = 25.312 [N] in direction of x positive