Question

Which of the following describes the transformations of g (x) = negative (2) Superscript x + 4 Baseline minus 2 from the parent function f (x) = 2 Superscript x?

shift 4 units left, reflect over the x-axis, shift 2 units down
shift 4 units left, reflect over the y-axis, shift 2 units down
shift 4 units right, reflect over the x-axis, shift 2 units down
shift 4 units right, reflect over the y-axis, shift 2 units down

Answer 1

A is correct

Explanation:

• Shift 4 units left, reflect over the x-axis, shift 2 units down

Answer 2

Answer: First option.

Explanation:

Below are some transformations for a function
`f(x)`:

1. If
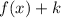`f(x)+k`, the function is shifted "k" units up.

2 If
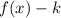`f(x)-k`, the function is shifted "k" units down.

3. If
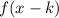`f(x-k)`, the function is shifted "k" units right.

4. If
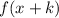`f(x+k)`, the function is shifted "k" units left.

5. If
`-f(x)`, the function is reflected over the x-axis.

6. If
`f(-x)`, the function is reflected over the y-axis.

Then, given the parent function
`f(x)`:

`f(x)=2^x`

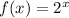
And knowing that the the other function is:

`g(x)=-2^((x+4))-2`

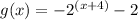
You can identify that the function
`g(x)` is obtained by:

- Shifting the function
`f(x)` 4 units left.

- Reflecting the function
`f(x)` 4 over the x-axis.

- Shifting the function
`f(x)` 2 units down.