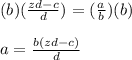Z=a/b+c/d solve for A

Question

asked Jul 14, 2021 71.1k views

1 Answer

Karo · Answer 1 · 2021-07-20T09:06:01+0000

Answer:
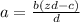
a=(b(zd-c))/(d)

Explanation:

Having the following equation given in the exercise:

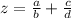
z=(a)/(b)+(c)/(d)

You can solve for "a" following this procedure:

1. You can apply the Subtraction property of equality and subtract
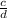(c)/(d) from both sides of the equation:

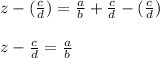
$z-((c)/(d))=(a)/(b)+(c)/(d)-((c)/(d))\\\\z-(c)/(d)=(a)/(b)$

2. Now you must subtract the terms on the left side of the equation. Notice that the Least Common Denominator is "d". Then:

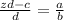
(zd-c)/(d)=(a)/(b)

3. Finally, you can apply the Multiplication property of equality and multiply both sides of the equation by "b". So, you get:

$(b)((zd-c)/(d))=((a)/(b))(b)\\\\a=(b(zd-c))/(d)$