Answer:
1120°
Explanation:
Use the sum of interior angles formula:
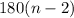
Where n is the number of sides.
In a nonagon, there are 9 sides, substitute 9 for n:
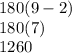
Since our nonagon is a regular nonagon, all 9 angles measure the same, so each angle measures:
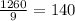
Subtract 140 from 1260:
