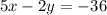Explanation:
The point-slope form of an equation of a line:

m - slope
(x₁, y₁) - point on a line
We have
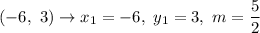
Substitute:

Convert to the slope-intercept form

m - slope
b - y-intercept
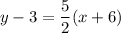 use the distributive property
use the distributive property
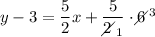
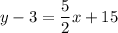 add 3 to both sides
add 3 to both sides

Convert to the standard form:
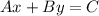
 multiply both sides by 2
multiply both sides by 2
 subtract 5x from both sides
subtract 5x from both sides
 change the signs
change the signs