Question

At a certain instant a particle is moving in the +x direction with momentum +8 kg m/s. During the next 0.13 seconds a constant force acts on the particle, with Fx=-7N and Fy= +5N.

What is the magnitude of the momentum of the particle at the end of this 0.13-second interval?

Answer 1

The momentum of the particle at the end of the 0.13 s time interval is 7.12 kg m/s

Step-by-step explanation:

The momentum of the particle is related to force by the following equation:

Δp = F · Δt

Where:

Δp = change in momentum = final momentum - initial momentum

F = constant force.

Δt = time interval.

Let´s calculate the x-component of the momentum after the 0.13 s:

final momentum - 8 kg m/s = -7 N · 0.13 s

final momentum = -7 kg m/s² · 0.13 s + 8 kg m/s

final momentum = 7.09 kg m/s

Now let´s calculate the y-component of the momentum vector after the 0.13 s. Since the particle wasn´t moving in the y-direction, the initial momentum in this direction is zero:

final momentum = 5 kg m/s² · 0.13 s

final momentum = 0.65 kg m/s

Then, the mometum vector will be as follows:

p = (7.09 kg m/s, 0.65 kg m/s)

The magnitude of this vector is calculated as follows:

`|p| = \sqrt{(7.09 kg m/s)^(2) + (0.65 kg m/s)^(2)} = 7.12 kg m/s`

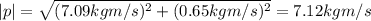
The momentum of the particle at the end of the 0.13 s time interval is 7.12 kg m/s