Question

Given right triangle QRS, what is the value of sin(30°)? StartFraction StartRoot 3 EndRoot Over 3 EndFraction One-half StartFraction StartRoot 3 EndRoot Over 2 EndFraction StartFraction 2 Over 1 EndFraction

Answer 1

B

Explanation:

Answer 2

Option 2:
`\sin(30)=(1)/(2)`

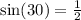
Explanation:

Given:

From the triangle shown below;

A triangle QRS with angle QRS = 90°, ∠QSR = 30°.

Side QR = 5, SQ = 10 and RS = 5√3

Now, we know from trigonometric ratio that,

`\sin (A) = (Opposite\ side)/(Hypotenuse)`

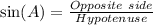
Here, opposite side of angle QSR is QR and Hypotenuse is the side opposite angle QRS which is SQ. Therefore,

`\sin(\angle QSR)=(QR)/(SQ)\\\\\\\sin(30)=(5)/(10)\\\\\\\sin(30)=(5)/(2* 5)=(1)/(2)`

Therefore, the value of sine of 30° is one-half. So, second option is correct.