Answer:
B_m = 3.186 x 10⁻³ T
Step-by-step explanation:
given,
diameter of the beam = 0.863 mm
Amplitude = 0.955 MV/m
speed of light = 2.99792 × 10⁸ m/s
he permeability of free space= 4π × 10⁻⁷ T· N/A
Amplitude of the magnetic field is given by

E_m is amplitude of the electric field.
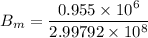
B_m = 0.31855 x 10⁻² T
B_m = 3.186 x 10⁻³ T
The amplitude of magnetic field is equal to 3.186 x 10⁻³ T