Question

A bucket of water with a mass of 2.0 kg is attached to a rope that is wound around a cylinder. The cylinder has a mass of 4.0 kg and is mounted horizontally on frictionless bearings. The bucket is released from rest. (a) Find its speed after it has fallen through a distance of 1.50 m

Answer 1

Step-by-step explanation:

Given mass of bucket is
`m=2\ kg`

mass of cylinder
`M=4\ kg`

Suppose T is the tension in the rope

For bucket
`mg-T=ma`

where a=acceleration

For cylinder with Radius R

`I* \alpha =T\cdot R`

`(MR^2)/(2)* (a)/(R)=T* R`

`T=(Ma)/(2)`

`a=(mg)/(m+0.5M)`

`a=4.9\ m/s^2`

Using
`v^2-u^2=2a s` for bucket

v=final velocity

u=initial velocity

s=displacement

`v^2-0=2* 4.9* 1.5`

`v=√(14.7)`

`v=3.83\ m/s`

Answer 2

The velocity of the bucket is 3.83 m/s.

Step-by-step explanation:

Given that,

Mass of bucket = 2.0 kg

Mass of cylinder =4.0 kg

Distance = 1.50 m

We need to calculate the speed

Using equation of motion

`v^2=u^2+2as`

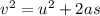
Put the value into the formula

`v^2=2* a*1.50`

`v=√(2* a*1.50)`

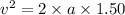
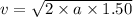
We need to calculate the tension

Using equilibrium equation

`mg=T+ma`

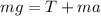
`T=m(g-a)`....(I)

Put the value into the formula

`T=2.0*(9.8-a)`

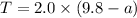
Now, using formula of tension

`T=I\\omega`...(II)

Moment of inertia of cylinder

`I=(1)/(2)MR^2`

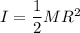
Angular velocity of cylinder is

`\omega=(a)/(R^2)`

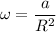
Put the value of angular velocity in equation (II)

`T=(1)/(2)M*((a)/(\omega))*\omega`

`T=(1)/(2)M* a`

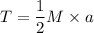
Put the value of tension in equation (I)

`(1)/(2)M* a=m(g-a)`

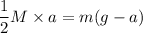
Put the value into the formula

`(1)/(2)*4.0* a=2.0(9.8-a)`

`a=(2.0*9.8)/(4.0)`

`a=4.9\ m/s^2`

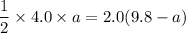
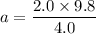
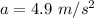
Put the value of acceleration in equation of motion

`v^2=2*4.9*1.50`

`v=√(2*4.9*1.50)`

`v=3.83\ m/s`

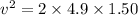
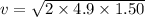
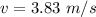
Hence, The velocity of the bucket is 3.83 m/s.