Question

Two wires are parallel, and one is directly above the other. Each has a length of 44.3 m and a mass per unit length of 0.0292 kg/m. However, the tension in wire A is 33.0 × 102 N, while the tension in wire B is 3.76 × 102 N. Transverse wave pulses are generated simultaneously, one at the left end of wire A and one at the right end of wire B. The pulses travel toward each other. How much time does it take until the pulses pass each other?

Answer 1

`t=0.0985\ s`

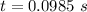
Step-by-step explanation:

Given:

• length of each wire,
  `l_a=l_b=44.3\ m`

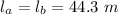
• linear mass density of each wire,
  `\mu_a=\mu_b=0.0292\ kg.m^(-1)`

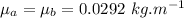
• tension in wire A,
  `T_a=3300\ N`

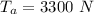
• tension in wire B,
  `T_a=376\ N`

Now, the velocity of the wave pulse in the stretched spring is given as:

FOR A:

`v_a=\sqrt{(T_a)/(\mu_a) }`

`v_a=\sqrt{(3300)/(0.0292) }`

`v_a=336.175\ m.s^(-1)`

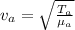
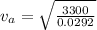
FOR B:

`v_b=\sqrt{(T_b)/(\mu_b) }`

`v_b=\sqrt{(376)/(0.0292) }`

`v_b=113.476\ m.s^(-1)`

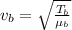
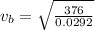
Let the distance at which the wave of A meets the wave of B be x from left then the distance from B is (44.3-x) meters.

Now the time taken is constant:

`t=(x)/(v_a) =(x-44.3)/(v_b)`

`(x)/(336.175) =(x-44.3)/(113.476)`

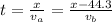
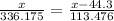
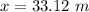`x=33.12\ m` is the distance travelled by pulse in wire A in when the pulse in the wire B meets

Now the time taken to travel this distance by pulse in wire A:

`t=(x)/(v_a)`

`t=(33.12)/(336.175)`

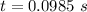`t=0.0985\ s` is the time taken by the two waves to pass each other.

Answer 2

0.09852 seconds

Step-by-step explanation:

`\mu` = Linear density = 0.0292 kg/m

`T_A` = Tesnsion in string A =
`33* 10^2\ N`

`T_B` = Tesnsion in string B =
`3.76* 10^2\ N`

t = Time taken till the pulses pass each other

Velocity of wave in a string is given by

`v_A=\sqrt{(T_A)/(\mu)}\\\Rightarrow v_A=\sqrt{(33* 10^2)/(0.0292)}`

`v_B=\sqrt{(T_2)/(\mu)}\\\Rightarrow v_B=\sqrt{(3.76* 10^2)/(0.0292)}`

`Distance=Speed* Time`

`L=(v_A+v_B)t\\\Rightarrow t=(L)/(v_A+v_B)\\\Rightarrow t=\frac{44.3}{\sqrt{(33* 10^2)/(0.0292)}+\sqrt{(3.76* 10^2)/(0.0292)}}\\\Rightarrow t=0.09852\ s`

The time taken is 0.09852 seconds