Answer:
The speed of the roller coaster at this point is 18.74 m/s.
Step-by-step explanation:
Given that,
Weight of the student, W = 655 kg
Weight of the roller coaster,
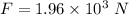
Radius of the roller coaster, r = 18 m
At the bottom of the loop, the weight of the roller coaster us given by :
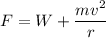
If m is the mass of the roller coaster,

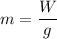
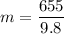
m = 66.83 kg
So,
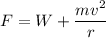
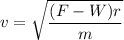
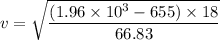
v = 18.74 m/s
So, the speed of the roller coaster at this point is 18.74 m/s. Hence, this is the required solution.