Question

All 423 Wisconsin public schools were all given a rating by the Wisconsin Department ofPublic Instruction based on several variables. The mean rating reported was 71.5 and the standard deviation was 4.87. To do a follow-up study a random sample of 40 schools was selected. In this sample, the mean rating was 70.9. So what is the probability of getting ,mean of 70.9.One of the researchers is alarmed, thinking the report may have been mistaken. Do you think this sample result is unusually low? Explain

Answer 1

`z = (\bar X -\mu)/(\sigma_(\bar X))= (70.9-71.5)/(0.77)=-0.779`

From this result we can conclude that the value of 70.9 is 0.78 deviation below the true mean of 71.5 and that can be considered as unusual. If we conduct a hypothesis test or a confidence interval we will see that we have enough evidence to conclude that the true mean is not significantly different from 71.5.

Explanation:

For this case from all the population we know that the population mean and deviation are:

`\mu = 71.5,\sigma = 4.87`

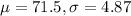
And we take a random sample of size n =40 and we got a sample mean calculated with the following formula:

`\bar X =(\sum_(i=1)^n X_i)/(n)= (\sum_(i=1)^(40) X_i)/(40)=70.9`

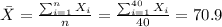
And we want to test if this value is unusually low.

Since the sample size is large n>30 we can use the central limit theorem who says that the distribution for the sample mean is given by:

`\bar X \sim N (\mu , (\sigma)/(√(n)))`

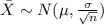
And on this case if we replace the values that we have we got:

`\bar X \sim N (\mu_(\bar X)=71.5,\sigma_(\bar X)= (\sigma)/(√(n))=(4.87)/(√(40))=0.77)`

For this case we can calculate how many deviations above or below is our calculated value from the sample of size 40, using the z score given by:

`z = (\bar X -\mu)/(\sigma_(\bar X))= (70.9-71.5)/(0.77)=-0.779`

From this result we can conclude that the value of 70.9 is 0.78 deviation below the true mean of 71.5 and that can be considered as unusual. If we conduct a hypothesis test or a confidence interval we will see that we have enough evidence to conclude that the true mean is not significantly different from 71.5.