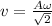Question

What is the magnitude of the velocity when the elastic potential energy is equal to the kinetic energy? (Assume that U=0 at equilibrium.)

Answer 1

Step-by-step explanation:

General Equation of SHM is given by

`x=A\cos \omega t`

`v=-A\omega \sin \omega t`

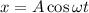
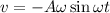
where x=position of particle

A=maximum Amplitude

`\omega =`angular frequency

t=time

At any time Total Energy is the sum of kinetic Energy and Elastic potential Energy i.e.
`(1)/(2)kA^2`

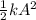
where k=spring constant

Potential Energy is given by
`U=(1)/(2)kx^2`

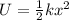
also it is given that Potential Energy(U) is equal to Kinetic Energy(K)

Total Energy
`=K+U`

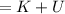
Total
`=2U=2* (1)/(2)kx^2`

`(1)/(2)kA^2=2* (1)/(2)kx^2`

`x=\pm (A)/(√(2))`

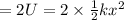
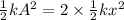
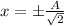
at
`x=(A)/(√(2))`

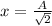
velocity is
`v=(A\omega)/(√(2))`