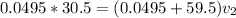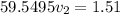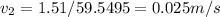Question

A 66.5-kg person throws a 0.0495-kg snowball forward with a ground speed of 30.5 m/s. A second person, with a mass of 59.5 kg, catches the snowball. Both people are on skates. The first person is initially moving forward with a speed of 2.20 m/s, and the second person is initially at rest. What are the velocities of the two people after the snowball is exchanged?

Answer 1

First person (66.5kg) has a speed of 2.18 m/s

Second person(59.5kg) has a speed of 0.025 m/s

Step-by-step explanation:

Assume the ball maintain constant speed of 30.5 m/s, neglecting air resistance. When the 1st person throw it, their momentum must be conserved before and after the throw

`(m + m_1)v_1 = m_1V_1 + mv`

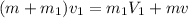
where m = 0.0495 kg, m1 = 66.5kg are the masses of the first person and the ball. v1 = 2.2m/s, V1, v = 30.5 m/s are the speed of the first person before, after, and the speed of the ball, respectively.

`(0.0495 + 66.5)2.2 = 66.5*V_1 + 0.0495*30.5`

`146.41 = 66.5V_1 + 1.51`

`V_1 = (146.41 - 1.51)/66.5 = 2.18 m/s`

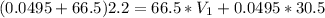
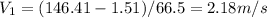
Similarly, the momentum for the 2nd person-ball system must be the same before and after the catch. Knowing that he is initially at rest:

`mv = (m+m_2)v_2`

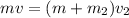
where m2 = 59.5, v2 are the mass the speed of the 2nd person after the catch:

`0.0495*30.5 = (0.0495 + 59.5)v_2`

`59.5495v_2=1.51`

`v_2 = 1.51 / 59.5495 = 0.025 m/s`