Question

Not going to lie my brain is dead

Answer 1

5² + 5² = x²

x = 7.1

x < 9

Step-by-step explanation:

Use pythagoras theorem on the square by doing 5^2 + 5^2 = x^2 an you get x^2 = 50. x = square root 50 which is 7.1. As x is less than the diameter of the circle it will fit in the circle without touching the edges

Answer 2

Length of diagonal of square is less than diameter of circle . Hence, the square will perfectly fit inside the circle without touching the edge of circle.

Step-by-step explanation:

Given:

Side length of square = S = 5 cm

Diameter of circle = D = 9 cm

To prove that the square with side length 5 cm will fit inside a circle with diameter 9 cm, without touching the edge of the circle.

A square has side length equal (similar length in all four sides). Diagonal of the square is greater than its side length and is the maximum dimension in case of a square.

So, if diagonal of square is less than diameter of circle; the square will fit inside the circle without touching its edges.

Let length of diagonal of square = L.

Using Pythagoras theorem to find L, .......................... (Refer attached figure)

`L^2 = S^2+S^2 = (5)^2 + (5)^2 = 25+25 = 50\\L=√(L^2) =√(50) = 7.07\ cm`

Since L=7.07 cm,
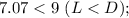`7.07<9\ (L<D);` Length of diagonal of square is less than diameter of circle.

The square will perfectly fit inside the circle without touching its edges.