Final answer:
The average velocity of the particle over the interval [7, 12] is 278 m/s. The estimated instantaneous velocity at t = 7, after rounding to the nearest whole number, is 148 m/s.
Step-by-step explanation:
Calculating Average Velocity and Estimating Instantaneous Velocity
To calculate the average velocity of a particle given by the position function
 over the time interval [7, 12], we'll use the formula
over the time interval [7, 12], we'll use the formula
Average velocity = ∆s/∆t = (s(12) - s(7))/(12 - 7)
First, we find s(12) and s(7):
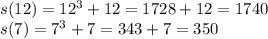
So, the average velocity = (1740 - 350)/5 = 1390/5 = 278 m/s.
For estimating the instantaneous velocity at t = 7, we find the derivative of the position function to obtain the velocity function:
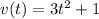
Then we substitute t = 7 into the velocity function:
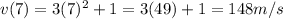
After rounding to the nearest whole number, the estimated instantaneous velocity at t = 7 is 148 m/s.